Understanding Prentice’s rule and its calculation.

What is Prentice’s rule in optics?

Prentice’s rule is a formula used in optics to calculate the prism power induced by a lens. It is commonly used in the dispensing of eyeglasses to patients who require prism correction for their vision.

PRENTICE’S RULE

prentice rule formula

The rule states that as the prismatic affect of a lens increases the further away the view point is from the optical center. The stronger the lens power, the more prism is induced into the lenses. The formula is written as

△ = hcm x D

△ = Prismatic Power

hcm = movement in cm (You will be given the movement in mm so do not forget to convert!)

D = dioptic power of the lens

Example of Prentice’s Rule using prentice rule formula

Example 1:

Prismatic Power (Δ) = 2 Movement (in mm) = 5

Converting movement to centimeters: Movement (in cm) = 5 mm / 10 = 0.5 cm

Now you can use the formula: △ = hcm x D

Substitute the values: 2Δ = 0.5 cm x D

Solving for D: D = 2Δ / 0.5 cm = 4 diopters/cm

So, the dioptic power of the lens is 4 diopters per centimeter.

Example 2:

Let’s say you have a lens with a prismatic power of 3Δ (diopters), and it shifts 8 mm when you look through it. What’s the movement in centimeters (hcm)?

Given: Prismatic Power (Δ) = 3 Movement (in mm) = 8

Converting movement to centimeters: Movement (in cm) = 8 mm / 10 = 0.8 cm

Using the formula: △ = hcm x D

Substitute the values: 3Δ = 0.8 cm x D

Solving for D: D = 3Δ / 0.8 cm = 3.75 diopters/cm

The dioptic power of the lens is 3.75 diopters per centimeter.

These examples demonstrate how the formulas △ = hcm x D and the conversion between millimeters and centimeters can help you calculate the prismatic power or dioptic power of a lens based on the observed movement.

How to find the best optometrist near me?

How is Prentice’s rule used in the field of optometry?

Prentice’s rule is used to calculate the amount of prism power needed to correct certain eye conditions and improve visual acuity in patients. Eye care professionals use this formula to prescribe eyeglasses with prism correction.

What is the formula of Prentice’s rule?

Prentice’s rule formula

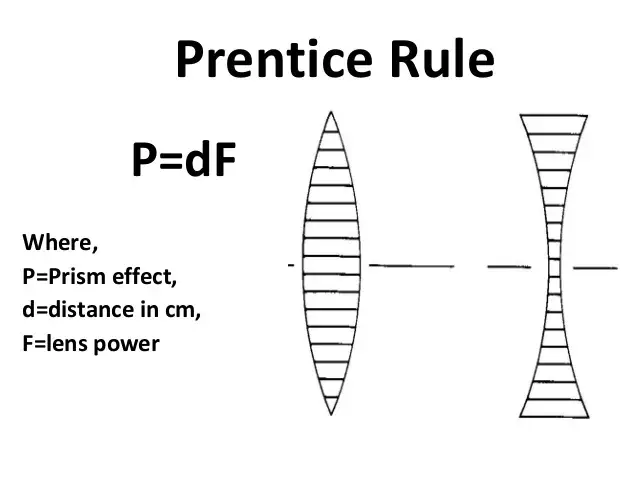
The formula for Prentice’s rule is:

P = d x h

where P is the prism power in prism diopters, d is the distance between the optical center of the lens and the prism base in meters, and h is the height of the prism from the base to the apex in meters.

For example, let’s say a patient requires a 2 prism diopter correction for their vision. The eye care professional determines that the prism base should be placed 15mm from the optical center of the lens, and the height of the prism is 10mm. Using Prentice’s rule, the prism power can be calculated as follows:

P = (15mm / 1000mm) x (10mm / 1000mm) = 0.15 x 0.01 = 0.0015 m^2

P = 2 diopters

Therefore, the lens should be prescribed with a 2 prism diopter correction.

What factors are considered when using Prentice’s rule?

The distance between the optical center of the lens and the prism base, as well as the height of the prism from the base to the apex, are the two factors considered when using Prentice’s rule.

Why is the direction of the prism base important in prism correction?

The direction of the prism base determines the direction in which light is bent. For example, a base-up prism would bend light upwards, while a base-in prism would bend light inwards towards the nose. It is important for eye care professionals to consider the direction of the prism base when prescribing prism correction.

Emmetropia Vs Ammetropia

Prentice’s rule problem using prentice’s rule formula

Prentice’s rule problem 1

A patient has an exotropia of 10 PD at distance and near. The distance between the patient’s eye and the optical center of the lens is 20 mm. What prism power is needed to correct the exotropia? (1)
Answer: P = d x delta = 20 mm x 10 PD = 2 prism diopters base-in

Problem 2

A patient has a vertical deviation of 4 PD in the right eye. The distance between the patient’s eye and the optical center of the lens is 15 mm. What prism power is needed to correct the vertical deviation? (2)
Answer: P = d x delta = 15 mm x 4 PD = 0.6 prism diopters base-down in the right eye

Problem 3

A patient has a horizontal deviation of 6 PD in the left eye. The distance between the patient’s eye and the optical center of the lens is 18 mm. What prism power is needed to correct the horizontal deviation?(3)
Answer: P = d x delta = 18 mm x 6 PD = 1.08 prism diopters base-in in the left eye

Problem 4

A patient with anisometropia has a vertical deviation of 2 PD in the right eye and 4 PD in the left eye. The distance between the patient’s right eye and the optical center of the right lens is 16 mm, and the distance between the patient’s left eye and the optical center of the left lens is 19 mm. What prism power is needed to correct the vertical deviation?(4)

Answer: For the right eye: P = d x delta = 16 mm x 2 PD = 0.32 prism diopters base-down. For the left eye: P = d x delta = 19 mm x 4 PD = 0.76 prism diopters base-down. Therefore, a prism of 0.32 prism diopters base-down in the right lens and 0.76 prism diopters base-down in the left lens is needed.

Problem 5

A patient has a horizontal deviation of 8 PD in the right eye and 2 PD in the left eye. The distance between the patient’s right eye and the optical center of the right lens is 17 mm, and the distance between the patient’s left eye and the optical center of the left lens is 16 mm. What prism power is needed to correct the horizontal deviation?(5)

Answer: For the right eye: P = d x delta = 17 mm x 8 PD = 1.36 prism diopters base-in. For the left eye: P = d x delta = 16 mm x 2 PD = 0.32 prism diopters base-out. Therefore, a prism of 1.36 prism diopters base-in in the right lens and0.32 prism diopters base-out in the left lens is needed.

Problem 6

A patient requires a 3 prism diopter correction. The prism base should be placed 12mm from the optical center of the lens, and the height of the prism is 8mm. Calculate the prism power using Prentice’s rule.
Answer: P = (12mm / 1000mm) x (8mm / 1000mm) = 0.096 x 3 = 0.288 diopters. Therefore, the lens should be prescribed with a 3 prism diopter correction.

Problem 7

A patient requires a 2 prism diopter correction. The prism base should be placed 10mm from the optical center of the lens, and the height of the prism is 6mm. Calculate the prism power using Prentice’s rule.
Answer: P = (10mm / 1000mm) x (6mm / 1000mm) = 0.06 x 2 = 0.12 diopters. Therefore, the lens should be prescribed with a 2 prism diopter correction.

Problem 8

A patient requires a 4 prism diopter correction. The prism base should be placed 18mm from the optical center of the lens, and the height of the prism is 12mm. Calculate the prism power using Prentice’s rule.
Answer: P = (18mm / 1000mm) x (12mm / 1000mm) = 0.216 x 4 = 0.864 diopters. Therefore, the lens should be prescribed with a 4 prism diopter correction.

Problem 9

A patient requires a 1 prism diopter correction. The prism base should be placed 8mm from the optical center of the lens, and the height of the prism is 5mm. Calculate the prism power using Prentice’s rule.
Answer: P = (8mm / 1000mm) x (5mm / 1000mm) = 0.04 x 1 = 0.04 diopters. Therefore, the lens should be prescribed with a 1 prism diopter correction.

Problem 10

A patient requires a 6 prism diopter correction. The prism base should be placed 20mm from the optical center of the lens, and the height of the prism is 15mm. Calculate the prism power using Prentice’s rule.
Answer: P = (20mm / 1000mm) x (15mm / 1000mm) = 0.3 x 6 = 1.8 diopters. Therefore, the lens should be prescribed with a 6 prism diopter correction.

Misconception about Prentice’s rule

1. Prentice’s rule states that the amount of prism required to correct a patient’s eye alignment is directly proportional to the distance between the optical center of the patient’s eyeglass lens and the patient’s eye.
   Answer: The formula for calculating prism power is P = d x delta, where P is the prism power, d is the distance between the optical center and the eye, and delta is the angle of deviation. Therefore, the amount of prism required increases as the distance between the optical center and the eye increases.(6)
   
2. Prentice’s rule applies to patients with a single ocular deviation, such as strabismus or amblyopia.
   Answer: Prentice’s rule can be used to calculate the prism power needed to correct a single ocular deviation, but it does not apply to patients with binocular vision problems or other complex visual disorders.(7)
   
3. Prentice’s rule can be used to calculate the amount of prism needed to correct vertical or horizontal deviations.
   Answer: Prentice’s rule can be used to calculate the prism power needed to correct both vertical and horizontal deviations. The formula is the same, but the distance between the optical center and the eye may be measured differently depending on the type of deviation.(8)
   
4. Prentice’s rule assumes that the patient’s eye is located at the optical center of the eyeglass lens.
   Answer: Prentice’s rule assumes that the patient’s eye is located at the optical center of the lens, but in practice, this is not always the case. Therefore, the rule should be used as a starting point, and adjustments may need to be made based on individual patient factors.(9)
   
5. Prentice’s rule can be used to calculate the prism power needed for a patient with anisometropia.
   Answer: Prentice’s rule can be used to calculate the prism power needed for a patient with anisometropia, but the measurement of the distance between the optical center and the eye must be done separately for each eye, as the distance may be different due to differences in refractive error.(10)
   

Reference:

1. Reference: Clinical Optics, 3rd ed., Chapter 8, p. 208.
2. Reference: Borish’s Clinical Refraction, 2nd ed., Chapter 14, p. 507.
3. Reference: Optics, Refraction and Contact Lenses, 2nd ed., Chapter 12, p. 215.
4. Reference: Clinical Optics, 3rd ed., Chapter 8, p. 209.
5. Reference: Borish’s Clinical Refraction, 2nd ed., Chapter 14, p. 508.
6. Reference: Borish’s Clinical Refraction, 2nd ed., Chapter 14, p. 507-508.
7. Reference: Clinical Optics, 3rd ed., Chapter 8, p. 207.
8. Reference: Optics, Refraction and Contact Lenses, 2nd ed., Chapter 12, p. 215.
9. Reference: Clinical Optics, 3rd ed., Chapter 8, p. 208.
10. Reference: Clinical Optics, 3rd ed., Chapter 8, p. 209.